by 
The publication “Improvements and Extension of the Linear Carbon Sink Model” shows that the global natural effective carbon sink depends on both the CO
concentration and the global (sea surface) temperature anomaly. A brief derivation of these relationships is provided below. In contrast to the cited publication, this investigation uses monthly deseasonalized CO2 concentration data 
, monthly global sea surface temperature data 
, and monthly emission data 
from interpolated yearly emission data at consecutive months 
.
The conservation of mass or the continuity equation imply that the monthly concentration growth
necessarily results from the sum of anthropogenic emissions and natural emissions 
, reduced by the monthly absorptions 
:
(1) 
By definition natural emissions here necessarily mean all CO2 emissions except the anthropogenic emissions.
The measurable sink effect
is the difference between anthropogenic emissions and the concentration growth 
: (2) 
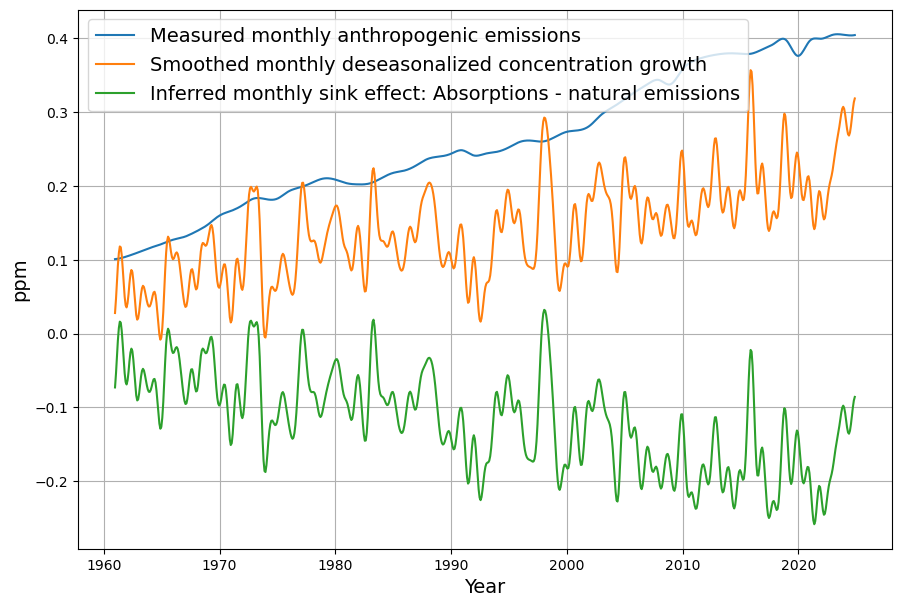
It follows directly from equation 1 that the directly measurable sink effect includes not only absorptions by the oceans and plants, but implicitly also natural emissions:
Therefore, the sink effect is not identical to the sum of all absorptions, but only the proportion of absorptions that have not been compensated by natural emissions during the current month.
The work “ Improvements and Extension of the Linear Carbon Sink Model ” shows that the global sink effect is represented described by an extended model of CO concentration and global sea surface temperature :
(3) 
As a matter of fact, the model fits best when there is a time lag of 6 months between temperature and sink effect.
The simple sink model described in the paper “Emissions and CO 2 Concentration—An Evidence Based Approach ”, which depends only on the CO2 oncentration, is used for comparison:
(4) 
Figure 2 shows that the simple model reproduces the trend very well, but that the fluctuations can only be described by the extended model, i.e., by including dependence on the sea surface temperature. The parameters for the best fit of the extended model with yearly data are 
, 
ppm/°C, 
ppm.
The increase in concentration can also be modeled using equations 2, 3, and 4 respectively:
(5) 
This is shown in Fig. 3. For comparison, the reconstruction with the simple sink model is shown here again (green curve).
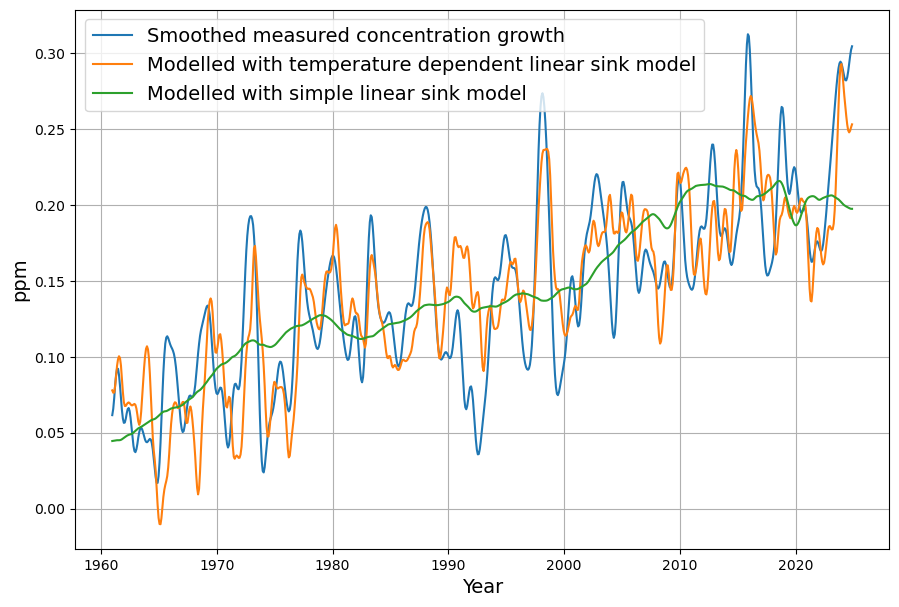
Reconstruction of concentration, differentiation of the influence of emissions and temperature
From the concentration growth, the course of the CO concentration can be reconstructed using the initial CO concentration , in this case the concentration in December 1958. According to equation 5, the concentration growth is controlled by the external state variables of anthropogenic emissions and temperature. Together with the determined parameter, the term results in the change in natural emissions due to the temperature anomaly . It is therefore interesting to first compare the magnitudes of both emission sources. The unit of measurement for emissions is GtC, which is calculated by multiplying the quantities otherwise measured in ppm by 2.123.
Figure 4 shows anthropogenic emissions since 1959 and natural emissions based on the sea surface temperature anomaly scale. Before 1975, temperatures and thus natural emissions, are predominantly negative. This is due to the arbitrary choice of the zero point of the anomaly temperature scale.
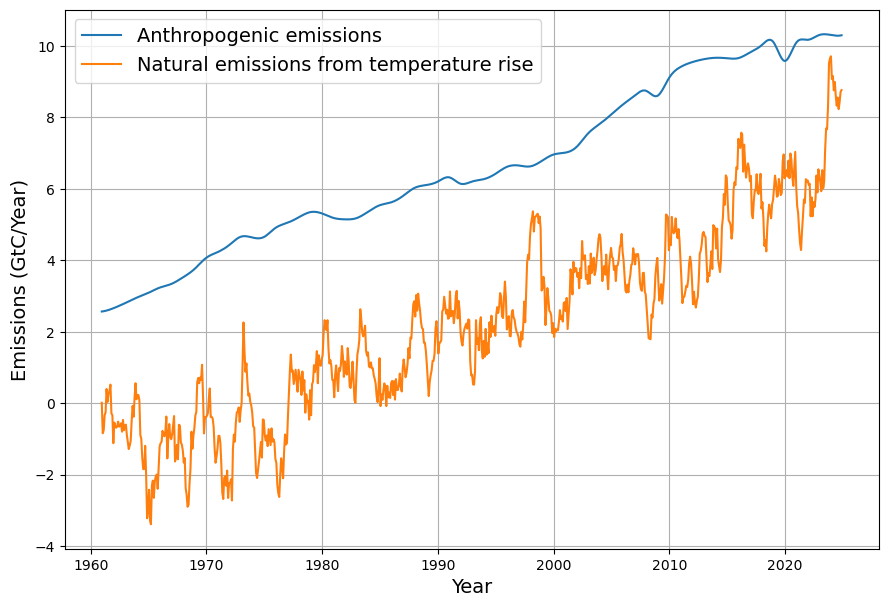
It is noticeable that anthropogenic emissions are on average about 4 GtC larger than the natural emissions. Overall, natural emissions are numerically much larger, since the constant term of about 30 GtC (14 ppm \cdot 2.123 GtC/ppm) also represents natural emissions. According to equation 14 in Improvements and Extension of the Linear Carbon Sink Model, these constant natural emissions define the equilibrium concentration at the temperature anomaly 0°C; with current figures, this equilibrium concentration is 315 ppm. This is not the pre-industrial state, which has a temperature anomaly of -0.48°C. Fig. 5 shows three selected model scenarios. In addition to the actually measured concentration values, the reconstruction with the actual temperature and emission trends (orange) is shown. As expected, this remains very close to the measured data.
In addition, two further scenarios are presented:
- Anthropogenic emissions remain at the 1959 level, and only the temperature continues to develop as we know it (green color). The CO2 concentration increases to about 370 ppm.
- The temperature remains at the 1959 level, but anthropogenic emissions continue to grow as usual. The concentration initially increases more steeply than if the temperature were also changing. This is because the temperature anomaly remains below zero until the mid-1970s. Only after 1983 does the resulting concentration remain below the reference value. Overall, anthropogenic emissions account for a larger share of the concentration increase than natural emissions, but in 2023, for example, the natural emissions share is very close to the anthropogenic emissions share.
It is also noticeable that the effects of both emission sources in terms of resulting concentration cannot simply be added together. The resulting concentration is lower than the sum of the concentrations of both emission components. This is due to the fact that absorption increases with increasing concentration. Both emission sources together have a smaller effect on the concentration than one would intuitively expect from their individual effects.
Is the temperature dependent rise in natural emissions consistent with the extended sink model?
We want to find out whether it is plausible that natural emissions rise at the rate of 3.2 ppm/°C per year? According to the publication “Temperature-associated increases in the global soil respiration record” during the 19 years from 1989 to 2008 the natural emissions from soil respiration 
have risen by 0.1 GtC per year, i.e. 1.9 GtC during the whole investigation period. During this time the global temperature has risen by 0.3°C. Therefore we have a temperature dependency of per yea
![\[\frac{\Delta R_S}{\Delta T}=\frac{1.9}{0.3} \text{ GtC/°C} = 6.33 \text{ GtC/°C}\]](https://klima-fakten.net/wp-content/ql-cache/quicklatex.com-5f7e9feaff9b668ee914c1426d197210_l3.png "Rendered by QuickLaTeX.com")
An
increase of 3.3 GtC/°C per year is reported by Hashimoto et al. According to Davidson et al there is considerable uncertainty in the determination of the temperature sensitivity of soil respiration.
Regarding the temperature dependence of the emissions from the oceans, we begin with the baseline of yearly emissions from oceans of 80-100 GtC according to the Global Carbon Budget. According to Takahashi et al. the relative change of CO partial pressure in seawater is 0.0423 per °C for a wide range of temperatures from 2°C to 28°C. Therefore the yearly increase in terms of absolute mass would be in the range between 
GtC/°C and 
GtC/°C
Adding the collected evidence for temperature dependency of soil respiration and ocean emissions results in a total range [3.3+3.4, 6.3+4.2]GtC/°C=[6.7,10.5]GtC/°C.
Conclusions
The extended sink model allows us to consider the effects of anthropogenic emissions and temperature increases on CO2 concentrations separately. This result contradicts those who believe that anthropogenic emissions fully explain the concentration changes since the beginning of industrialization.
The extended sink model also contradicts those who claim that, due to the large turnover of the natural carbon cycle, anthropogenic emissions play no role at all. It is actually trivial that anthropogenic emissions as a direct input must necessarily have an effect. The truth is that both factors have an influence of a similar order of magnitude, although anthropogenic emissions are slightly more predominant.
